Duels in the town of Discretion are rarely fatal. There, each contestant comes at a random moment between \(5\) AM and \(6\) AM on the appointed day and leaves exactly \(5\) minutes later, honor served, unless his opponent arrives within the time interval and then they fight. What fraction of duels lead to violence?
Let \(X\) be the arrival time of the first contestant, and \(Y\) that of the second. For simplicity, we will scale the duration between \(5\) AM and \(6\) AM to the value \(1\), so that \(\frac{1}{12}\) corresponds to \(5\) minutes. Using this notation, the fraction of duels that will to violence can be stated as:
\[P\left(|X - Y| < \frac{1}{12}\right) = P\left(-\frac{1}{12} < X - Y < \frac{1}{12}\right)\]
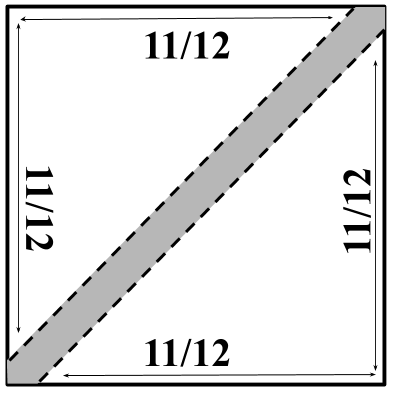
Graphing this inequality gives the following shaded region representing duels that lead to violence:

The relevant region is a unit square (since \(0 \leq X \leq 1\) and \(0 \leq Y \leq 1\)), and \(X\) and \(Y\) are both uniformly distributed, so we can calculate the probability of violence as the total area of the square, minus the areas that do not lead to violence, which gives \(1 - \left(\frac{11}{12}\right)^2 = \frac{23}{144} \approx \frac{1}{6}\).